Change in Stars--Interactive Text

Earth Science Extras

by Russ Colson

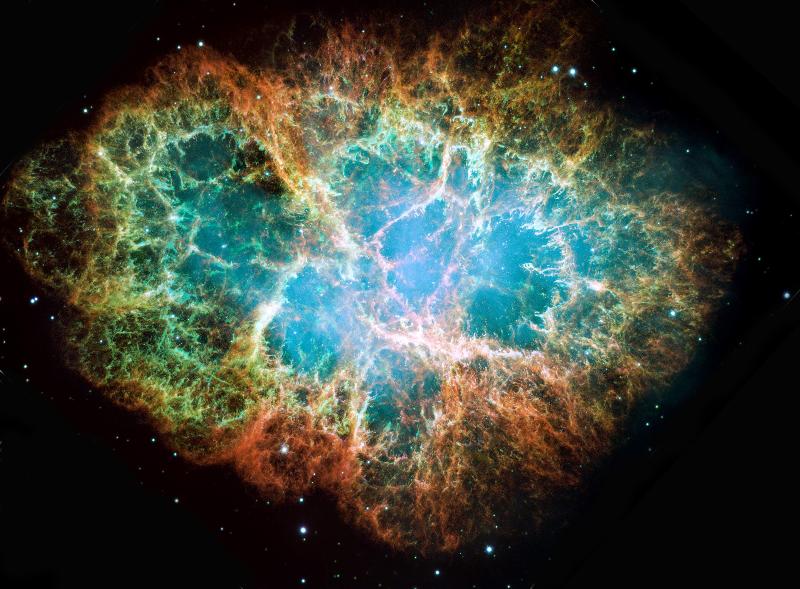
Crab Nebula, The Hubble Space Telescope, StSci-PRCos-37.

The Crab Nebula is the remnant of a supernova that was observed in human history in 1054AD. One account from a Chinese astronomer is the following: "I humbly observe that a guest star has appeared; above the star there is a feeble yellow glimmer... it had appeared at dawn, in the direction of the east, under the watch of Tiānguān (天關, Zeta Tauri). It had been seen in daylight, like Venus. It had rays stemming in all directions, and its colour was reddish white. Altogether visible (during the day?) for 23 days."

It's also possible that the supernovae was observed by North American Pueblo people and recorded in a pictograph (Brandt, J. C. & Williamson, R. A. "The 1054 Supernova and Native American Rock Art" (1979), Journal for the History of Astronomy, Archaeoastronomy Supplement, Vol. 10, p.S1). As of this writing, you can find a picture of the pictograph at https://www2.hao.ucar.edu/Education/SolarAstronomy/supernova-pictograph

Recap of Lecture

Value: 2

Objects 'glow' by blackbody radiation ("blackbody" refering to the idea that when we consider blackbody radiation we are only talking about radiation emitted from a body, not radiation that is reflected). Comparing the sun to the Earth:

a. Both Earth and the sun are emitting blackbody radiation, the sun as visible light, the Earth in wavelengths too long for the human eye to see

b. Both Earth and the sun are emitting blackbody radiation, the sun as visible light, the Earth in wavelengths too short for the human eye to see

c. Although the sun is 'glowing' by blackbody radiation, the Earth is not, as can be seen by the fact that it gets dark at night when the sun is not shining on the surface of Earth

d. The Earth is glowing by blackbody radiation in the infrared part of the electromagnetic spectrum, but the sun is not glowing, but rather emitting nuclear radiation energy.

Value: 2

Value: 3

The star classification of Father Angelo Secchi does not quite match the later and more sophisiticated classification of Annie Jump Cannon, however we can somewhat match the up a few of their overlapping categories. You can do this based on the lecture.

The task is to match the lettered items with the correct numbered items. Appearing below is a list of lettered items. Following that is a list of numbered items. Each numbered item is followed by a drop-down. Select the letter in the drop down that best matches the numbered item with the lettered alternatives.

a. Cannon category A star: high temperature star
b. Cannon category M star: lower temperature star
c. Cannon category G star: medium temperature star

1. Secchi Class I: Blue-white stars with heavy H and metallic lines
2. Secchi Class II: Yellow stars with less strong H lines but with metallic lines
3. Secchi Class III: Orange stars with complex band spectra

Value: 2

The Life Cycle of Stars--A literature review investigation

One of the most important aspects of learning science is to do scientific investigations. An investigation involves making observations in the natural world, identifying a question you want to examine further, making more focussed observations and doing experiments, organizing the experimental and observational data into graphs, tables, and illustrations, interpreting the data, inferring a model that explains the observations, testing the model against new observations, explaining the model to others, and arguing from evidence that the model provides a reasonable and correct interpretation of reality.

All of this requires a lot of time and mentoring and is hard-to-impossible to do in an on-line class.

However, a different type of investigation is where you investigate scientific ideas that other people have already figured out and explained--we might call it a literature review investigation or even an "online" investigation-- this type of investigation, although not a scientific investigation, also has value in the world. It's also valuable to gain practice in figuring out the difference between reliable information online and less reliable information (or sometimes, even complete junk). We are going to do an investigation of this sort.

Learn all you can about the life cycle of stars. We will be considering in particular 1) stars perhaps slightly larger than our sun and 2) stars much larger than our sun whose ultimate destiny is to go out in a supernova. Key search words to help you find important info might be stellar evolution (or star life cycle) and Hertzsprung-Russell diagram.

When you think you are ready, test yourself against the two questions below; the first qustion is for smaller stars perhaps a bit bigger than our sun and the second for bigger supernova-destined stars. There are a lot of pieces to these questioins so don't rush through them. There are some complexities to the lifecycle of stars dependent on exact size and chemical composition, so the illustrations below have been simplified to illustrate key changes and transitions.

Value: 17

For Sun-sized stars or a little bigger: Match the position on the evolutionary path of the star with the processes happening at that location. Remember, the "Y" axis portrays the brightness of the star, the "X" axis the temperature (hotter to the left).

a. Fusion of Hydrogen (H) outside the star's core causes the star to expand and cool
b. Eventually, aftter more time than the age of the universe, the white dwarf is expected to cool to a black dwarf star (not at all the same thing as a black hole!)
c. Nebula/gas cloud collapses under the force of gravity, heating up from gravitational energy and growing brighter.
d. Red Giant Stage
e. 2nd Red Giant stage. Pulses of H and He fusion in diffferent layers of the star cause brightening and dimming.
f. Nebula/gas cloud collapses under the force of gravity, growing smaller and dimmer
g. Helium (He) fuel in the core of the star is exhausted. He fusion in layers outside the core commences. Star cools and explands.
h. Interior fusion in the star ends,. Collapsing of the star causes an increase in Temperature so that even as the star shrinks, the brightness stays roughly constant. Outer layers of the star are ejected forming what is called a planetary nebula--although it is neither a planet nor a true nebula.
i. Hydrogen (H) fuel supply in the core of the star is exhausted.
j. Nebula/gas cloud becomes hot enough and compressed enough that Hydrogen (H) fusion to form Helium (He) starts in the core. Pressure from gravity and radiation force from the nuclear fusion are in balance. Nucleosynthesis notes: Example reaction
k. Fusion of Helium (He) is occuring in the Star's Core to form Carbon (C) Nucleosynthesis notes: Example reactions
l. White dwarf star--with no more fuel for fusion, the star cools and dims.

1. A
2. B
3. C
4. D
5. E
6. F
7. G
8. H
9. I
10. J
11. K
12. L

Value: 8

For bigger Stars: Match the position on the evolutionary path of the star with the processes happening at that location. Remember, the "Y" axis portrays the brightness of the star, the "X" axis the temperature (hotter to the left).

a. Nebula/gases collapsing under the force of gravity, growing denser, smaller, and dimmer
b. Hydrogen (H) in the core is exhausted and H fusion shifts to areas outside the core
c. Red Supergiant. Helium (He) fusion in the core of the star making Carbon (C)
d. Star grows hot and compressed enough that Hydrogen (H) fusion begins in the core, making He.
e. Blue supergiant. Fusion of different materials at different levels in the star, like layers of an onion. Heavier elements are fused at deeper levels. Nucleosynthesis notes. Examples of alpha-process reactions (alpha particles are like He nuclei) and so on, for big stars it can go all the way to Because Fe is the most stable nucleus, further energy-generating fusion is not possible and fusion will end.
f. Hydrogen (H) fusion in outer layers causes the star to expand, accompanied by cooling.
g. Fusion comes ot an end, the star goes through a complex process of collapsing that ends in a supernovae
h. Nebula/gases collapsing under the force of gravity, heating up and staying the same brightness or only slightly brighter as the increase in temperature is balanced by the decrease in size.

1. A
2. B
3. C
4. D
5. E
6. F
7. G
8. H

Nucleosynthesis refers to the process of making elements (synthesizing nuclei), and is a major consideration in the life cycle of stars, which you probably encountered in your online researches. The major process that often shows up in the study of the life cycle of stars can be simplified as the "alpha process", basically adding an alpha particle to elements to create heavier elements, by reactions such as those shown below (an alpha particle = 2 protons and 2 neutrons = the nucleus of a He atom). For elements lighter than Argon, the reactions below are a composite, and the actual mechanism for these reactions is thought to involve fusion of C with C and O with O, and thus be more complex.

equation image indicator

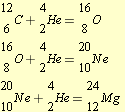
and so on up to equation image indicator

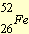
In the notation above, which you probably also encountered in your online researches, the letter is the symbol for an element (e.g. C = carbon). The number in the upper left is the atomic mass = number of protons plus number of neutrons. The number in the lower left atomic number = the number of protons (which defines an element--carbon always has 6 protons). Notice that each successive element formed by this process has 2 more protons and 2 more neutrons, making the atome mass increase in increments of 4.

This process can't make elements heavier than Iron (Fe). Heavier elements, and elements whose protons and neutrons don't increase in even steps of 2. must be formed by other processes. Many heavier elements are probably formed in supernovae (you may have encountered the idea of 'r' processes in your online searches, where a rapid flux of neutrons, such as might be generated by a supernovae, create heavier elements and isotopes).

Thus, nearly all the elements of our solar system--nearly all the elements in your body--were made in the hearts of stars. We really are all made of stardust!

Value: 2

Figuring out the Life Cycle of Stars

Spectroscopy, newly possible in the 1800's, opened the door to learning about star temperature and what stars are made of. However, the thrill of classifying stars was found in its potential to provide insight into how stars change through time. To a significant extent, the life-cycle pathways for different sizes of stars which we examined in the lessons above were based on mathematical calculations of rates of nuclear reactions, temperature, pressure and brightness of stars inferred from a theoretical understanding of nuclear physics. However, in science, theoretical modeling must be based on, tested by, and calibrated against observations in the real world. For the life cycle of stars, these observations include the proportions of different star types that we see in the observable universe.

We can figure out the lifecycle of stars, not by watching one star go through its life cycle, but rather by observing groups of stars in different life stages.

Conceptual introduction:

Suppose that you have a tube, like the one shown below. Every two minutes you poke a marble in one end and a marble pops out the other (steady state...same number of marbles in the tube and same distribution of marbles in each zone). You can see how the distribution of marbles in the tube allows one to estimate how long a marble spends in a particular part of the tube.

Value: 2

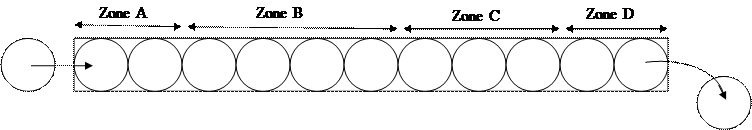
Applying the "marbles in the tube" illlustration to human populations

Suppose you arrived on earth and noticed three different types of human, which you call small, large, and slow. You watch for two years, and observe that one of the slow humans dies each year (you infer those must be old humans) and one small human is born each year (you infer those must be the young humans) Suppose that this population has reached a steady state, that is, each year one death is balanced by one birth, and that the proportions of each type of human is as shown below.

Determine the time spent at each stage of life, in years.

Of course, people are more complicated than this, not always maintaining a steady state population (that's what baby booms are about) and not always living exactly the same length of time (not for lack of trying). Stars are also more complicated. It took more than a century after Jesuit priest Angelo Secchi began classifying stars to figure out the life cycle of stars.

Applying the "marbles in a tube" illustration to a case where steady state has not yet been reached:

Consider a slightly more complicate version of the marbles-in-a-tube puzzle. Again assume that a new marble is inserted every 2 minutes. It's clear that steady state has not been reached in the case below because the system has not been continuing long enough to reach the end (in nature this can only be realized if you have some means of determining the what the end truly is, like the death of a star by a known or anticipated process). Even though you don't have a steady state, you can still infer a number of things.

Value: 2

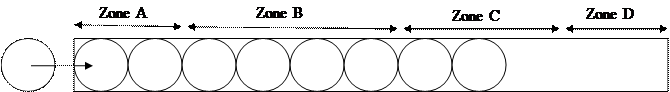
Applying the "marbles in a tube" illustration to a simulated study of the life cycle of a sun-like star

The puzzles below use simulated data, simplified to consider only stars of the same mass or age. There are many more types of stars than discussed below, which made the real puzzle much more challenging. Tens of thousands of studies, some taking entire careers, were needed to solve the puzzle of stellar evolution.

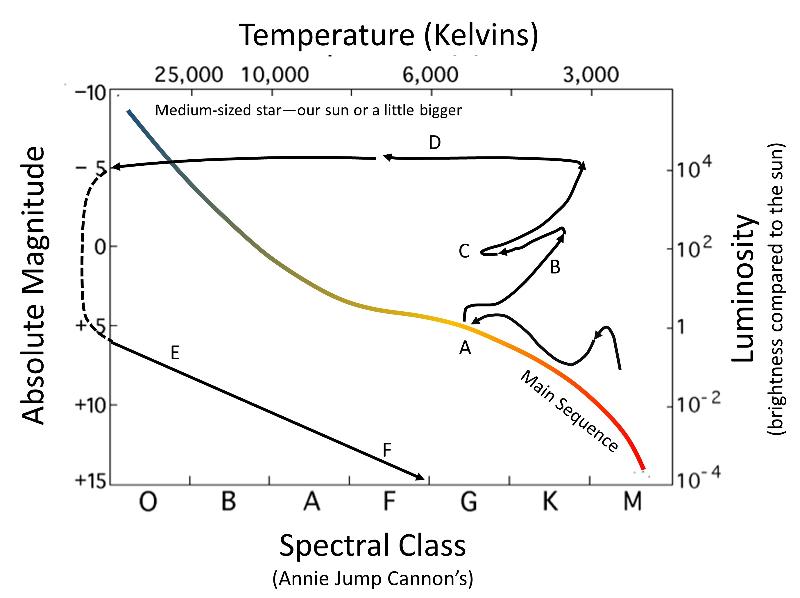
Suppose we looked at stars with the same mass and chemical composition characteristics as our Sun (or a little bigger). We pick a set of stars that we think might represent a population in which new stars have been born somewhat regularly over a long period of time (steady state at least for stages that have been completed in the population of stars). For the population of stars we have chosen, we find the distribution of stars as given below.

A: There are 1 million sun-like stars, burning H in their core, with a new one born every 9 thousand years

B: There are 111 thousand red giants, burning H in a shell outside of the core

C: There are 11000 yellow giant stars burning He in their core

D: There is 1 planetary nebula star

E: There are 900 thousand white dwarf stars

F: There are no black dwarf stars

Reverse Data Simulation of the Life Cycle of Larger Stars

Large stars go through a much different, and shorter, life cycle ending in a supernova instead of a white dwarf. For example, a star with a mass more than 18x our sun, such as a star that went supernova in 1987 in a nearby galaxy called the Large Magellanic Cloud, is believed to have the life cycle as listed below:

Inferred life cycle of SN-1987A type II supernova

1) entered Main Sequence 11 million years ago

2) left Main Sequence 700,000 years ago (becoming a Red Supergiant)

3) Began He fusion in core 650,000 years ago (Red and Blue supergiant)

4) He fuel exhausted 45,000 years ago

4) Began Carbon fusion 12,000 years ago

5) Supernova in 1987.

Now, figure out how many stars might be in each of the stages below in a galaxy the size of the Milky Way, using the life cycle data above, and from the knowledge that, based on Chinese star records, there have been perhaps 20 supernova in the Milky Way galaxy over the past 2000 years (the last one visible to the naked eye occuring in 1604AD).

Value: 2

An Age of Star Cluster Puzzle

As seen in the models for the life cycle of stars above, bigger stars are much shorter-lived than smaller stars. A star cluster is a group of stars that were all 'born' about the same time. By looking at what stage of life different stars in the cluster are in, we can figure out the age of the star cluster.

Value: 3

A couple of spectrum puzzles

Remember from the lecture that absorption and emission lines from specific elements are superimposed on a star's overall blackbody radiation signature. Lines are specific to elements, but also dependent on temperature. It is on the basis of the spectral lines that Annie Jump Cannon developed the star classification that we use today.

Value: 2

The black body radiation spectrum of a star can tell us its temperature. Below is the spectrum of our Sun (with absorption and emission lines smoothed out). Based on black body radiation, estimate the temperature of the surface of the Sun. (To interpolate the peak wavelength accurately, you may need to convert the lower scale to logarithms. Also, don't be sloppy--use a ruler).

Here is a reminder of how logarithms work:

1m = 100cm = 1000mm = 1,000,000μm = 1,000,000,000nm = 10,000,000,000Å

log 10²= log 100 = 2; log 10³ = log 1000 = 3; log 10000 = 4, etc. log 10^2.5 = log 316 = 2.5

The X axis tic marks on either side of the peak are the following:

10³Å = 10²nm

1um= 10³nm

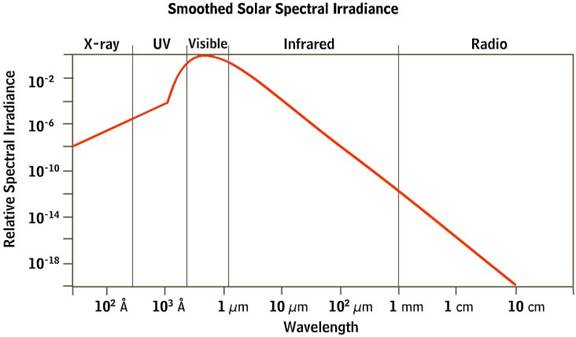
hon 314 solar spectrum smoothed

Image source unknown.

Value: 2

last updated 4/23//2020. The image of the Crab Nebula comes courtesy of NASA, ESA, J. Hester. Images whose source is not known are indicated in the text. Other text and pictures are the property of Russ Colson.